[DOC]http://www.encyclopediaofmath.org/index.php/Dirac_quantization
A qualitative discussion of a successful theory of ...
www.winnerscience.com/wp.../08/bcs-theory-of-superconductivity.doc
Missing: dirac
Winner Science | BCS Theory of Superconductivity
www.winnerscience.com/science/bcs-theory-of-superconductivity/
Missing: dirac
List of Nobel laureates in Physics - Wikipedia, the free ...
en.wikipedia.org/wiki/List_of_Nobel_laureates_in_Physics
Wikipedia
Loading...
Physicochemical properties of metal nanoparticle by shreya ...
www.slideshare.net/.../physicochemical-properties-of-metal-nanoparticle-...
Missing: dirac
Dirac quantization
From Encyclopedia of Mathematics
canonical quantization
A term referring to a proceeding that associates to a commutative algebra of physical observables, of a classical mechanical system, a non-commutative algebra of linear operators on a suitable Hilbert space (or, more generally, on a locally convex topological vector space; cf. also Linear operator). Such a proceeding, called canonical quantization, has been first mathematically axiomatized by P.A.M. Dirac [a5] (which justifies the name). Subsequently, many other contributions have been given to generalize this concept in a geometrical way, by obtaining constructive representations of commutative algebras characterizing differential manifolds in non-commutative algebras. The most remarkable examples are geometric quantization (B. Kostant and J.M. Souriau [a11], [a26], [a30]) and deformation quantization (F. Bayen, M.V. Karasev, M. Flato, C. Fronsdal, A. Lichnerowicz, D. Sternheimer, and V.P. Maslov [a32], [a33], [a6], [a10]). These coincide for non-relativistic systems of a finite number of particles with the (Dirac) canonical quantization.
So, "Dirac quantization" can be used also as synonymous of "canonical quantization" . However, nowadays (2000) the term "Dirac quantizations" means quantizations of partial differential equations that not necessarily coincide with canonical quantizations. For an example, see the Crumeyrolle–Prástaro quantizations of partial differential equations [a17], [a18], [a19], [a20]. Furthermore, for Lagrangian field theories, an approach of functional type, called the Feynman path method, has had a big success. In fact, this allows one to obtain approximated descriptions of electroweak nuclear phenomena, where the perturbative methods can be of practical convenience. However, the Feynman path method is, in general, not well mathematically founded, as it requires integration on infinite-dimensional manifolds. In some sense, this aspect has been improved in the framework of gauge theory, as the quotient with respect to gauge groups produces finite-dimensional manifolds [a2], [a34], [a35], [a36], [a37], [a7], [a8], [a9]. (A lot of recent mathematical studies are in some sense related to such a point of view and have given new interesting prospects in pure mathematics. See e.g. [a31].) Moreover, the Feynman path method is related to the so-called covariant quantization, which prescribes the quantum bracket
for the operators 
corresponding to the local components 
of a field 
, "localized" at the point 
of the space-time 
:
where 
is the propagator of the theory [a12].
This approach is essentially related to the Peierls bracket [a16], but has many limitations and inconsistencies from the mathematical point of view. In fact, first of all it refers to linear dynamic equations of variational type; furthermore, it does not work well for chiral fields, i.e., fields that are sections of non-vector bundles (see Quantum field theory). Any attempt to extend such proceedings to theories described by means of non-linear and non-Lagrangian partial differential equations did fail, until some recent geometric studies on the quantization of partial differential equations [a17], [a18], [a19], [a20]. More precisely, in [a17], [a18], [a19], [a20] the concept of formal Dirac quantization of partial differential equations is introduced, that is, roughly speaking, a procedure that associates a measure space (quantum situs) to a partial differential equation. This quantization becomes effective if on (the classic limit of) the quantum situs one recognizes (pre-)spectral measures (quantum spectral measures of partial differential equations).
The axiomatization of the concept of (Dirac) quantization of a classical system, represented by a partial differential equation
, can be given on the ground of mathematical logic by means of algebra homomorphisms 
, where 
is the logic of 
, that is the Boolean algebra of subsets of the classic limit 
of the quantum situs 
of 
(in other words, 
is the set of solutions of 
), and 
is a quantum logic, that is, an algebra of (self-adjoint) operators on a locally convex topological vector (Hilbert) space 
(cf. also Hilbert space; Locally convex space; Self-adjoint operator): 
. This is equivalent to the assignment of pre-spectral measures on 
: 
[a17], [a18], [a19], [a20], [a38].
In this way it is possible to give a generalization of the concept of covariant quantization in the general framework of the geometric theory of partial differential equations. (Of course, there are many effective quantizations, but the most interesting from the physical point of view is the covariant quantization or the canonical quantization, that is, the covariant quantization observed by a physical frame.) In fact, in that geometric context, it is proved that any physical observable deforms the original partial differential equation around a classical solution. In this way one can associate to the Lie algebra of classical observables a non-commutative algebra, i.e., the quantum algebra of the system, defined by means of the bracket
for any two observables 
, 
, at the solution-section 
of 
. Here, 
are operator-valued distributions, at the section 
, on a locally convex topological vector space 
, depending on 
, and 
is a distributive kernel, which generalizes the usual concept of propagator made for linear differential operators [a4], [a12], and which is canonically associated to the non-linear dynamic equation of the theory at the section 
[a17], [a18], [a19], [a20].
In [a17], [a18], [a19], [a20], a geometric interpretation of the concept of propagator for non-linear partial differential equations is given. This is related to the concept of (integral) bordism [a21], [a22], [a23]. In this way the quantization of partial differential equations is connected to this important sector of algebraic topology, introduced by R. Thom and L.S. Pontryagin [a1], [a15], [a27], [a28]. This geometric approach justifies in some sense the belief that "quantization" is synonymous of "deformation" (see e.g., [a32], [a33], [a6], [a10] and also the modern concept of quantum geometry in [a3], [a14], [a29]). More recently (1990s), A. Prástaro has generalized the concept of Dirac quantizations for partial differential equations also to non-commutative (quantum) partial differential equations, i.e., partial differential equations built in the category of quantum manifolds (see [a20], [a24], [a25]). In this way one gets a mathematically well-founded geometric theory of quantum partial differential equations that is useful to formulate a quantum field theory unifying gravity and electromagnetic forces with nuclear forces. See also the algebraic categorial formulation of quantizations on Hopf algebras given by V. Lychagin [a13] (cf. also Hopf algebra). Since the quantum group is formulated in the language of Hopf algebras (cf. also Quantum groups), many formal quantum theories are given in the framework of such an algebra. However, there is also a more structural geometric reason that emphasizes this algebra. In fact, in [a21], [a22], [a23], [a24], [a25] it is proved that on the space of all conservation laws of a (quantum) partial differential equation the structure of (quantum) Hopf algebra can be recognized.
A term referring to a proceeding that associates to a commutative algebra of physical observables, of a classical mechanical system, a non-commutative algebra of linear operators on a suitable Hilbert space (or, more generally, on a locally convex topological vector space; cf. also Linear operator). Such a proceeding, called canonical quantization, has been first mathematically axiomatized by P.A.M. Dirac [a5] (which justifies the name). Subsequently, many other contributions have been given to generalize this concept in a geometrical way, by obtaining constructive representations of commutative algebras characterizing differential manifolds in non-commutative algebras. The most remarkable examples are geometric quantization (B. Kostant and J.M. Souriau [a11], [a26], [a30]) and deformation quantization (F. Bayen, M.V. Karasev, M. Flato, C. Fronsdal, A. Lichnerowicz, D. Sternheimer, and V.P. Maslov [a32], [a33], [a6], [a10]). These coincide for non-relativistic systems of a finite number of particles with the (Dirac) canonical quantization.
So, "Dirac quantization" can be used also as synonymous of "canonical quantization" . However, nowadays (2000) the term "Dirac quantizations" means quantizations of partial differential equations that not necessarily coincide with canonical quantizations. For an example, see the Crumeyrolle–Prástaro quantizations of partial differential equations [a17], [a18], [a19], [a20]. Furthermore, for Lagrangian field theories, an approach of functional type, called the Feynman path method, has had a big success. In fact, this allows one to obtain approximated descriptions of electroweak nuclear phenomena, where the perturbative methods can be of practical convenience. However, the Feynman path method is, in general, not well mathematically founded, as it requires integration on infinite-dimensional manifolds. In some sense, this aspect has been improved in the framework of gauge theory, as the quotient with respect to gauge groups produces finite-dimensional manifolds [a2], [a34], [a35], [a36], [a37], [a7], [a8], [a9]. (A lot of recent mathematical studies are in some sense related to such a point of view and have given new interesting prospects in pure mathematics. See e.g. [a31].) Moreover, the Feynman path method is related to the so-called covariant quantization, which prescribes the quantum bracket
for the operators corresponding to the local components of a field , "localized" at the point of the space-time :  |
is the propagator of the theory [a12]. This approach is essentially related to the Peierls bracket [a16], but has many limitations and inconsistencies from the mathematical point of view. In fact, first of all it refers to linear dynamic equations of variational type; furthermore, it does not work well for chiral fields, i.e., fields that are sections of non-vector bundles (see Quantum field theory). Any attempt to extend such proceedings to theories described by means of non-linear and non-Lagrangian partial differential equations did fail, until some recent geometric studies on the quantization of partial differential equations [a17], [a18], [a19], [a20]. More precisely, in [a17], [a18], [a19], [a20] the concept of formal Dirac quantization of partial differential equations is introduced, that is, roughly speaking, a procedure that associates a measure space (quantum situs) to a partial differential equation. This quantization becomes effective if on (the classic limit of) the quantum situs one recognizes (pre-)spectral measures (quantum spectral measures of partial differential equations).
The axiomatization of the concept of (Dirac) quantization of a classical system, represented by a partial differential equation
, can be given on the ground of mathematical logic by means of algebra homomorphisms , where is the logic of , that is the Boolean algebra of subsets of the classic limit of the quantum situs of (in other words, is the set of solutions of ), and is a quantum logic, that is, an algebra of (self-adjoint) operators on a locally convex topological vector (Hilbert) space (cf. also Hilbert space; Locally convex space; Self-adjoint operator): . This is equivalent to the assignment of pre-spectral measures on : [a17], [a18], [a19], [a20], [a38]. In this way it is possible to give a generalization of the concept of covariant quantization in the general framework of the geometric theory of partial differential equations. (Of course, there are many effective quantizations, but the most interesting from the physical point of view is the covariant quantization or the canonical quantization, that is, the covariant quantization observed by a physical frame.) In fact, in that geometric context, it is proved that any physical observable deforms the original partial differential equation around a classical solution. In this way one can associate to the Lie algebra of classical observables a non-commutative algebra, i.e., the quantum algebra of the system, defined by means of the bracket
 |
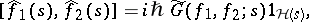, , at the solution-section of . Here, are operator-valued distributions, at the section , on a locally convex topological vector space , depending on , and is a distributive kernel, which generalizes the usual concept of propagator made for linear differential operators [a4], [a12], and which is canonically associated to the non-linear dynamic equation of the theory at the section [a17], [a18], [a19], [a20]. In [a17], [a18], [a19], [a20], a geometric interpretation of the concept of propagator for non-linear partial differential equations is given. This is related to the concept of (integral) bordism [a21], [a22], [a23]. In this way the quantization of partial differential equations is connected to this important sector of algebraic topology, introduced by R. Thom and L.S. Pontryagin [a1], [a15], [a27], [a28]. This geometric approach justifies in some sense the belief that "quantization" is synonymous of "deformation" (see e.g., [a32], [a33], [a6], [a10] and also the modern concept of quantum geometry in [a3], [a14], [a29]). More recently (1990s), A. Prástaro has generalized the concept of Dirac quantizations for partial differential equations also to non-commutative (quantum) partial differential equations, i.e., partial differential equations built in the category of quantum manifolds (see [a20], [a24], [a25]). In this way one gets a mathematically well-founded geometric theory of quantum partial differential equations that is useful to formulate a quantum field theory unifying gravity and electromagnetic forces with nuclear forces. See also the algebraic categorial formulation of quantizations on Hopf algebras given by V. Lychagin [a13] (cf. also Hopf algebra). Since the quantum group is formulated in the language of Hopf algebras (cf. also Quantum groups), many formal quantum theories are given in the framework of such an algebra. However, there is also a more structural geometric reason that emphasizes this algebra. In fact, in [a21], [a22], [a23], [a24], [a25] it is proved that on the space of all conservation laws of a (quantum) partial differential equation the structure of (quantum) Hopf algebra can be recognized.
No comments:
Post a Comment