| 積分符號∫中間加壹個圈∮是什麼意思? 在閉曲線上的曲線積分,我們常常在積分號上面寫壹個○以強調,即把積分號∫寫成∮。 注意:在閉曲線上的曲線積分可以寫∮,也可以仍然寫∫,但不是閉曲線上的曲線積分,不可以用∮,只能寫∫。 在曲面積分裡也有類似的情形。 iask.sina.com.cn/b/609...&retcode=0 數學符號表 zh.wikipedia.org/wiki/...7%E8%A1%A8 zh.wikipedia.org/wiki/...F%E5%88%86 什麼是路徑積分? 就是曲線積分,可以用來求曲線的長度 例如 有 y=f(x),現在要 求 f(x) 在 x 屬於[a,b]中 f(x) 的長度,就可以表示為 Integrate[Sqrt[f'[x]^2+1],{x,a,b}] f(x)的導函數的平方+1後開方在 x 屬於[a,b]上的定積分 en.wikipedia.org/wiki/Line_integral In mathematics, a line integral (sometimes called a path integral, contour integral, curve integral, or curvilinear integral; not to be confused with calculating arc length using integration) is an integral where the function to be integrated is evaluated along a curve. The function to be integrated may be a scalar field or a vector field. The value of the line integral is the sum of values of the field at all points on the curve, weighted by some scalar function on the curve (commonly arc length or, for a vector field, the scalar product of the vector field with a differential vector in the curve). This weighting distinguishes the line integral from simpler integrals defined on intervals. Many simple formulae in physics (for example, W=F·s) have natural continuous analogs in terms of line integrals (W=∫C F· ds). The line integral finds the work done on an object moving through an electric or gravitational field, for example. en.wikipedia.org/wiki/Line_integral 在數學中,曲線積分或路徑積分是積分的壹種。積分函數的取值沿的不是區間,而是特定的曲線,稱為積分路徑。曲線積分有很多種類,當積分路徑為閉合曲線時,稱為環路積分或圍道積分。 在曲線積分中,被積的函數可以是標量函數或向量函數。積分的值是路徑各點上的函數值乘上相應的權重(壹般是弧長,在積分函數是向量函數時,壹般是函數值與曲線微元向量的標量積)後的黎曼和。帶有權重是曲線積分與壹般區間上的積分的主要不同點。物理學中的許多簡單的公式(比如說W=\vec F\cdot\vec d)在推廣之後都是以曲線積分的形式出現(W=\int_C \vec F\cdot d\vec s)。曲線積分在物理學中是很重要的工具,例如計算電場或重力場中的做功,或量子力學中計算粒子出現的概率。 en.wikipedia.org/wiki/Line_integral 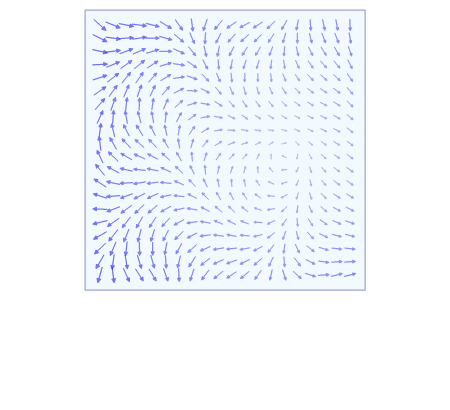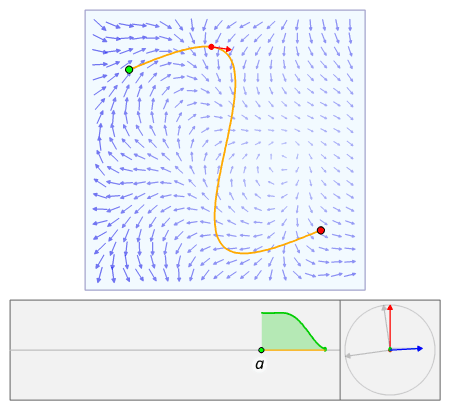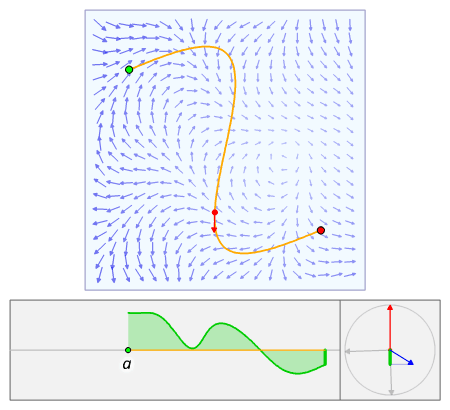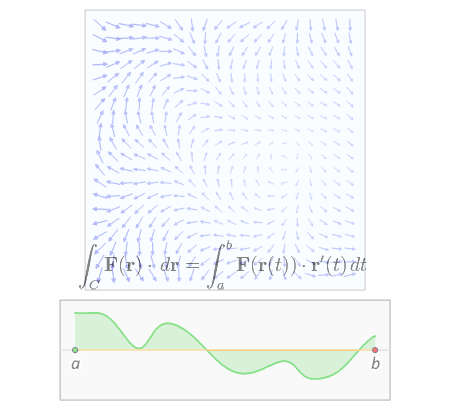 zh.wikipedia.org/wiki/...F%E5%88%86  曲線積分和曲面積分 www.math.pku.edu.cn:80...oad/28.pdf 曲線積分 web.tongji.edu.cn/~mat.../z1/z1.htm jpkc.scezju.com/upload...dex2_1.htm 123.125.115.53/view/1001256.htm see also: www.tieku001.com/474502/1.html 費曼圖是美國著名物理學家、繼薛定諤和海森柏後提出第叁種建立量子力學的方式的理查德 費曼所創立的壹種用形象化的方法方便地處理量子場中各種粒子相互作用的圖。 zh.wikipedia.org/wiki/...C%E5%9B%BE 文盲正侃時間史 www.tianya.cn/publicfo...1009.shtml www.tianya.cn/publicfo...7438.shtml [more] jpkc.hbut.edu.cn/lxy/d...book_3.pdf jpkc.hbut.edu.cn/lxy/d...book_2.pdf jpkc.hbut.edu.cn/lxy/d...book_1.pdf | |
| 樓主 |
Monday, June 16, 2014
曲線積分或路徑積分是積分的壹種。積分函數的取值沿的不是區間,而是特定的曲線,稱為積分路徑
Subscribe to:
Post Comments (Atom)
No comments:
Post a Comment