Introduction to Supersymmetry
20th century physics has seen two major paradigm shifts in the way we understand Mother Nature. One is quantum mechanics, and the other is relativity. The marriage between the two, called quantum field theory, conceived an enfant terrible, namely anti-matter. As a result, the number of elementary particles doubled. We believe that 21st century physics is aimed at yet another level of marriage, this time between quantum mechanics and general relativity, Einstein's theory of gravity. The couple has not been getting along very well, resulting in mathematical inconsistencies, meaningless infinities, and negative probabilities. The key to success may be in supersymmetry, which doubles the number of particles once more. Why was anti-matter needed? One reason was to solve a crisis in the 19th century physics of classical electromagnetism. An electron is, to the best of our knowledge, a point particle. Namely, it has no size, yet an electric charge. A charged particle inevitably produces an electric potential around it, and it also feels the potential created by itself. This leads to an infinite "self-energy" of the electron. In other words, it takes substantial energy to "pack" all the charge of an electron into small size. On the other hand, Einstein's famous equation
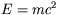says that
mass of a particle determines the energy of the particle at rest. For an
electron, its rest energy is known to be 0.511 MeV. For this given amount of
energy, it cannot afford to "pack" itself into a size smaller than the size of a
nucleus. Classical theory of electromagnetism is not a consistent theory below
this distance. However, it is known that the electron is at least ten
thousand times smaller than that.
(b)
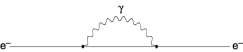(c) 
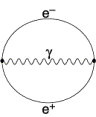
What saved the crisis was the existence of anti-matter, positron. In quantum mechanics, it is possible to "borrow" energy within the time interval allowed by the uncertainty principle. Once there exists anti-matter, which can annihilate matter or be created with matter, what we consider to be an empty vacuum undergoes a fluctuation to produce a pair of electron and positron together with photon, annihilating back to vacuum within the time interval allowed by the uncertainty principle (a). In addition to the effect of the electric potential on itself (b), the electron can annihilate with a positron in the fluctuation, leaving the electon originally in the fluctuation to materialize as a real electron (c). It turns out, these two contributions to the energy of the electron almost nearly cancel with each other. The small size of the electron was made consistent with electromagnetism thanks to quantum mechanics and the existence of anti-matter.
Currently
the Standard Model of particle physics is facing a similar crisis. We know that
our Universe is filled with a mysterious condensate of Higgs boson, which
disturbs matter particles and forces, not letting them go far and hence making
them massive. For example, the carrier of the weak force, W boson, bumps on the
Higgs condensate all the time, and the force has become short-ranged, extending
only over a thoughsandth of the size of nuclei. All masses of known elementary
particles must have come from the Higgs boson. However, the mass of the Higgs
boson receives a large contribution from its interaction with itself making it
impossible for us to study physics at smaller distances. Because the gravity is
believed to be unified with other forces at an extremely small distance called
Planck length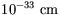 
, the marriage between quantum mechanics and
gravity appears a remote dream.
Supersymmetry is an idea that history repeats itself to solve similar
problems. For every particle, there is a superpartner whose spin differs by 1/2.
By doubling the number of particles again, there is similar cancellation between
the process with ordinary particles only and another process with their
superpartners. Then the Standard Model can describe physics down to the Planck
length, making the marriage a realistic hope. In fact, it is a necessary
ingredient in the only available candidate for quantum theory of gravity, string
theory.
(b)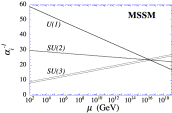
Supersymmetry actually makes the unification of three other forces, strong, weak, and electromagnetic, also a reality. In (a), in the Standard Model without supersymmetry, the strengths of three forces change as a function of energies, and become closer to each other at very high energies. Together with supersymmetry (Minimal Supersymmetric Standard Model or MSSM), however, they become equal within a percent-level accuracy. Where are superpartners? It is a realistic hope that coming accelerator experiments will find them, possibly Tevatron collider at Fermilab, Illinois, or the Large Hadron Collider at CERN, Geneva, Switzerland in this decade.
(b)
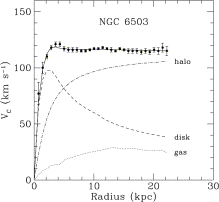
It is amusing that superpartners may actually be everywhere without us noticing. Our galaxy is known to be full of Dark Matter, weakly interacting particles whose gravitational pull binds the galaxy together despite its fast rotation. The picture (a) shows the measurement of Doppler shift in 21cm line that allows us to determine the rotational speed of other galaxies. The rotational speed is much faster than what the gravitional pull by stars would allow (b). One of the best candidates for Dark Matter is the lightest supersymmetric particle. Even though supersymmetry solves many problems in particle physics, it also poses new problems.
- What makes superpartners heavier than ordinary particles? This is the problem of supersymmetry breaking.
- Why are superpartners so well hidden in rare phenomena? Arbitrary mass spectrum of superpartners actually would cause too large effects in rare processes that change flavor of particles. There must be some special reason why such effects are well hidden.
- How do we discover superpartners experimentally? How do we extract information on the mechanism of supersymmetry breaking?
- How does supersymmetry impact cosmology? Is the lightest supersymmetric partner the Dark Matter? How do we prove it?
Our group had made substantial contributions to the theoretical study of supersymmetry. It was Bruno Zumino, together with Julius Wess, who discovered the possibility of supersymmetry in four-dimensional spacetime back in 1973. Until early 1980's, however, it was more of a mathematical curiosity than a serious possibility for the realistic theory of nature. Lawrence Hall, together with Joe Lykken and Steven Weinberg, laid the foundation of relatistic supersymmetric phenomenology. Mary K Gaillard made it possible to systematically study quantum effects in supersymmetric theory of gravity, supergravity. Hitoshi Murayama, together with Gian Giudice and two former Berkeley postdocs, Markus Luty and Riccardo Rattazzi, found subtle quantum contributions to masses of superpartners, independently with two other former Berkeley postdocs, Lisa Randall and Raman Sundrum. This home page is based on the introduction in Supersymmetry Phenomenology by Hitoshi Murayama.
-
murayama@physics.berkeley.edu
- Phone (510) 486 5589, Campus phone (510) 642-1019
- Location Bldg. 50A 5109 (LBL), 447 Birge (Campus)
No comments:
Post a Comment