§2
经典函数与量子算符的Weyl对应_CNKI学问
经典函数与量子算符的Weyl对应--《大学物理》2012年04期 - 知网空间
www.cnki.com.cn/.../CJFDTOTAL-DXWL201204001.htm - 轉為繁體網頁
Page 1 Page 2 研究会報告 重力場の粒子生成に伴う揺動的反作用" 京 ...
由 森川雅博 著作 - 1987
この問題の本質は, 単に c数・ q数の区別だけでなく, 一様なバッ ク グラン. ドから構造が形成されるメ カニズムであ り) 宇宙論に特有の問題ではない。 実際, イ ンフ レーシ ョ ...孟沥原的量子力学史 Part26_水银_新浪博客
blog.sina.com.cn/s/blog_49806bcc0100cxpt.html - 轉為繁體網頁
c stood for "classical," while q stood for "quantum"; but later Dirac suggested that they respectively stood for "commutative" and "queer."[63]
| Chapter XII Queer Numbers | ||||
Quantum Algebra
Dirac's successful adaptation of the canonical methods of classical dynamics depended much on his conception of "quantum algebra." Several of the symbolic operations which he performed on quantum variables were, indeed, meaningless from the point of view of Göttingen's authorities. For instance, as Jordan explained in a letter to Dirac, there could be
― 320 ―
no matrix (even a continuous one) representing an angle variable, since there is no conjugate operator to operators like the action variables, which have a discrete spectrum and no accumulation point.[62]
q-Numbers
In "The fundamental equations" Dirac had adopted Heisenberg's original definition of quantum variables as arrays of ordinary numbers, and also the interpretation of the polarization matrix as giving radiation intensities. In his next article he adopted a more abstract stance. The quantum variables were "magnitudes of a kind that one cannot specify explicitly." They had to be defined only by the fundamental equations which they obeyed, while their representation in terms of infinite matrices, if any existed , had to be deduced from these equations. To capture the essence of his position in one word, Dirac introduced the "q -numbers," which were defined by their algebraic properties alone: they could be added and multiplied as in a ring; only some of them commuted with all other q -numbers, in which case they were called "c -numbers." Apparently, c stood for "classical," while q stood for "quantum"; but later Dirac suggested that they respectively stood for "commutative" and "queer."[63]
In a spectacular illustration of his strategy, Dirac subsequently derived the existence of a matrix representation for most q -numbers in the case of multiperiodic systems.[64] He first modified his definition of quantum action-angle variables in such a way that they no longer presupposed matrices. Just as in the classical theory, (w, J ) would be action-angle variables if and only if the Hamiltonian was a function of J only, and any q -number (save the multiple-valued ones) could be expressed in the form[65]

Consider now two q -numbers x and y and their product xy . We have
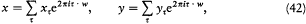
― 321 ―
and
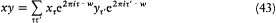
or
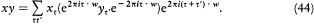
In order to transform the latter expression we first prove the identity

which is valid for any function f expressible as a power series of J . The relation of commutation

implies
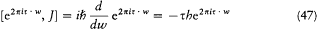
or

Equating the nth powers of the two members of the latter equation produces
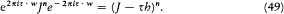
Then, linearly superposing powers of J and composing the results justifies the identity (45) for power series.
The expression (44) for the product xy now becomes
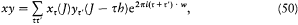
or

For the sake of transparency change the notation Ct (J ) into C (J, J -t h). Then
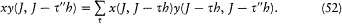
This symbolic relation, noticed Dirac, becomes a matrix product as soon as the J 's are given c -number values nh (i.e., Jr= nr h). Therefore, any q -number may be represented by a matrix qmn , wherein n and m refer
― 322 ―
to two possible values of the action variables J . The action-variables J themselves and the energy H (J ) are represented by diagonal matrices with diagonal elements corresponding to J = nh . Naturally, the different values of J are assumed to characterize stationary states in Bohr's sense.
Thus defined, the matrices do not yet exhibit the time dependence implied by the fundamental relation

Dirac remedied this by studying the time derivative of the quantum Fourier exponentials:
Through the identity (45) this transforms into
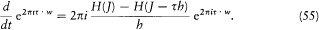
Taking the derivative of a q -number with respect to time therefore amounts to multiplying its C (J, J -t h) by 2p i times the Bohr frequency D t H/h. In this magic way Dirac recovered Heisenberg's matrix form and Bohr's frequency condition.[66]
Dirac still had to show that the polarization matrix in this scheme provided transition probabilities, as originally asserted by Heisenberg. He did this in the following manner. The harmonic development of the quantum electric polarization P is essentially ambiguous, for it can be written in two equally justified forms:
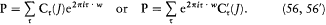
According to identity (45), however, the coefficients Ct and
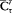 are related by are related by
This shows that Ct (J ) is naturally connected to two stationary states, J = nh , and J = (n -t )h , whereas it was connected only to one stationary state in the classical Fourier development. This suggests, in conformity with Bohr's postulates, that radiation is related to a transition
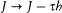
― 323 ―
between two stationary states and that the matrix C (J, J -t h) represents the amplitude of the oscillations connected with this transition.[67]
This reasoning of Dirac's reflected a strategy reminiscent of Eddington's principle of identification. It first introduced abstract entities defined only by their mutual relations, the q -numbers, then developed the formal consequences of these relations in such a way as to suggest an identification of their physical meaning. There were, however, some differences. According to Eddington, the primitive relations were dictated by the mind, whereas Dirac obtained them through the classical analogy or, better, through some kind of "extensive abstraction" of the structure of Hamiltonian dynamics. Moreover, the identification of observable quantities was not completely dictated by the mind; it relied on Bohr's postulates and also on the privileging of action-angle variables, which was a remnant of the old form of the correspondence principle.
A Mathematical Digression
The essence of Dirac's approach was to leave the properties of q -numbers open to the needs of future developments that might occur in quantum mechanics. Nevertheless, his interest in the purely mathematical side of his theory led him to introduce supplementary axioms that would enrich the algebra of q -numbers and make it closer to the algebras which he already knew, namely, quaternions and Baker's symbols. For instance, he occasionally admitted that all q -numbers had inverses, and he excluded divisors of zero (i.e., numbers such that qq ' = 0 with
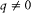 and and 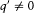 ). In a mathematical paper of 1926 he added another axiom that was supposed to be necessary for a proper definition of q -number functions: for any two q -numbers x and y there had to exist a q -number b such that y = bxb-1 .[68] ). In a mathematical paper of 1926 he added another axiom that was supposed to be necessary for a proper definition of q -number functions: for any two q -numbers x and y there had to exist a q -number b such that y = bxb-1 .[68]
As Léon Brillouin noted in a letter to Dirac, none of these axioms was suited to quantum variables. An operator introduced by Pauli in 1926, the spin-raising operator S+ = Sx + iS y , furnishes a simple counterexample to the two first axioms. It divides zero since
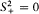 , and it cannot be inverted since a relation S+q = 1 leads to an absurdity once multiplied by S+ on the left. Finally, if the last axiom were true, any two quantum variables would have the same spectrum—patently untrue. The algebraic , and it cannot be inverted since a relation S+q = 1 leads to an absurdity once multiplied by S+ on the left. Finally, if the last axiom were true, any two quantum variables would have the same spectrum—patently untrue. The algebraic
― 324 ―
properties of q -numbers, Brillouin concluded, could not differ from those of arbitrary matrices.[69]
Fortunately, Dirac's attempts to axiomatize the q -numbers did not interfere with their practical use. Despite Brillouin's claim, the q -numbers proved to be more general than Heisenberg's original matrices, since they could cover both discrete and continuous spectra and allowed quantum angle variables that had no matrix representation, and since their applicability was not limited to stationary systems, as exemplified in the calculation of the Compton effect. Above all, Dirac wanted flexibility:
Dirac thus set forth a general program by which arbitrary physical situations might be analyzed with q -numbers, the properties of the q -numbers being tailored to fit the physical situations as well as the fundamental equations.
Stagnation
In May 1926 Dirac put together in his dissertation the first fruits of his conception of quantum mechanics. By then he had found nearly all that could be learned from the q -number adaptation of the method of uniformizing variables. There were obvious signs that the magic of this method was being exhausted. Even a problem that was simply treated on the basis of the old quantum theory, the H-atom, received a fairly complicated treatment within the q -number theory, regardless of the high mathematical skills deployed. The very problem that motivated Heisenberg's discovery of matrix mechanics, the calculation of the intensities of hydrogen lines, was no more accessible to Dirac than it was to the Göttingen group.
― 325 ―
In general (with the questionable exception of the Compton effect), Dirac's methods could not be used to treat more problems than the old quantum theory, precisely because they were nothing but a noncommutative reformulation—should we say complication?—of the methods of this theory.
There was a more fundamental obstacle which Dirac disclosed in the late spring of 1926, either before or right after his first use of the Schrödinger equation: if, in the spirit of Heisenberg's theory, matrices refer only to observable processes, there cannot be any action-angle representation of them in the case of atoms with more than one electron. Let indeed m and n be two similar quantum numbers referring to two electrons in a given atom. According to a natural extension of Heisenberg's observability principle, stationary states differing only by a permutation of m and n should be identified, since there is no observable difference between the transitions
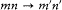 and and 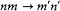 . Consider now the Fourier exponential e2p i(2t · w) corresponding to the transition . Consider now the Fourier exponential e2p i(2t · w) corresponding to the transition 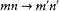 . Then the Fourier exponential e2 p i(2t · w) corresponds to a transition . Then the Fourier exponential e2 p i(2t · w) corresponds to a transition 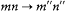 , with , with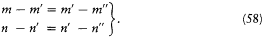
If m'n ' is to be identified with n'm ', one might as well have written
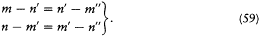
Here comes the absurdity: the values of m"n " deduced from each system cannot refer to the same stationary state since they are neither identical nor related through a permutation. With this ingenious argument Dirac closed a first chapter of his involvement in the history of quantum mechanics.[71]
|
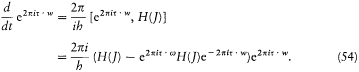
No comments:
Post a Comment