Jounce

From Wikipedia, the free encyclopedia

Jump to: navigation, search

In physics, jounce or snap^[1] is the fourth derivative of the position vector with respect to time, with the first, second, and third derivatives being velocity, acceleration, and jerk, respectively; in other words, the jounce is the rate of change of the jerk with respect to time. Jounce is defined by any of the following equivalent expressions:

$\vec s =\frac {d \vec j} {dt}=\frac {d^2 \vec a} {dt^2}=\frac {d^3 \vec v} {dt^3}=\frac {d^4 \vec r} {dt^4}$

the following equation is used for constant jounce.

$j = j. + st$
$a = a. + j.t + \frac{1}{2}st^2$
$v = v. + a.t + \frac{1}{2}j.t^2 + \frac{1}{6}st^3$
$S = v.t + \frac{1}{2}a.t^2 + \frac{1}{6}j.t^3 + \frac{1}{24}st^4$

where

$\vec s$ is constant jounce,

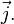$\vec j.$ is initial jerk,

$\vec j$ is final jerk,

$\vec a.$ is initial acceleration,

$\vec a$ is final acceleration,

$\vec v.$ is initial velocity,

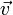$\vec v$ is final velocity,

$\vec S$ is distance or displacement

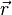$\vec r$ is position,

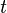$\mathit{t}$ is time.

The notation $\vec s$ (used in ^[2]) is not to be confused with the displacement vector commonly denoted similarly. Currently, there are no well-accepted designations for the derivatives of jounce. The fourth, fifth and sixth derivatives of position as a function of time are "sometimes somewhat facetiously"^[2]^[3] referred to as "Snap", "Crackle", and "Pop" respectively.
The dimensions of jounce are distance per (time to the power of 4). In SI units, this is "metres per quartic second", "metres per second per second per second per second", m/s⁴, m · s^-4

phymath999

Monday, July 22, 2013

In physics, jounce or snap[1] is the fourth derivative of the position vector with respect to time, with the first, second, and third derivatives being velocity, acceleration, and jerk, respectively

Jounce

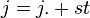
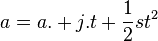
No comments:

Post a Comment