Friday, July 12, 2013

phymath01 mass of a system in special relativity is the norm of its energy-momentum four vector; otherwise, it is mc.

mass of a system in special relativity is the norm of its energy-momentum four vector; otherwise, it is $mc$ .

We define the Euclidean norm $\vert a\vert$ of a vector $a=(a_1,a_2)\in R^2$ as

$\vert a\vert =(a_1^2+a_2^2)^{1/2}$ .

By Pythagoras theorem, the Euclidean norm $\vert a\vert$ of the vector $a=(a_1,a_2)$ is equal to the length of the hypothenuse of the right angled triangle with sides $a_1$ and $a_2$ . In other words, the Euclidean norm Euclidean norm of the vector $a=(a_1,a_2)$ is equal to the distance from the origin to the point $a=(a_1,a_2)$ , or simply the length of the arrow $(a_1,a_2)$ .

The norm $\vert a\vert$ of a vector $a=(a_1,a_2)$ is $\vert a\vert=(a_1^2+a_2^2)^{1/2}$ .
We have

$\vert \lambda a\vert =\vert\lambda\vert \vert a\vert$ if $\lambda\in R$ and $a\in R^2$ ;

multiplying a vector by the real number $\lambda$ changes the norm of the vector by the factor $\vert\lambda\vert$ .
The zero vector $(0,0)$ has Euclidean norm 0 and if a vector has Euclidean norm 0 then it must be the zero vector.
The Euclidean norm of a vector measures the “length” or “size” of the vector.
There are many possible ways to measure the “size” of a vector corresponding to using different norms. We will meet several alternative norms of a vector $a=(a_1,a_2)$ below, such as $\vert a_1\vert +\vert a_2\vert$ or $\max(\vert a_1\vert, \vert a_2\vert )$ . We used $\vert a_1\vert +\vert a_2\vert$ in the definition of Lipschitz continuity of $f: R^2\rightarrow R$ above.
Example: If $a=(3,4)$ then $\vert a\vert =\sqrt{9+16}=5$ , and $\vert 2a\vert =10$ .

廣義相對論中的質量[编辑]

维基百科，自由的百科全书

跳转至：导航、搜索

显示▼隐藏▲ 查论编廣義相對論

入門、數學表述、验证

基礎概念	狭义相对论、等效原理、世界线、黎曼几何

现象	开普勒问题、参考系拖拽、引力时间延迟、测地线效应、引力透镜效应、重力波、黑洞、事件視界、引力奇点

方程	線性化重力、後牛頓形式論、爱因斯坦场方程、弗里德曼方程

進階理論	卡魯扎-克萊因理論、量子引力

方程解答	史瓦西度規、凱斯納度規、克爾度規、克尔-纽曼度规、萊斯納-諾德斯特洛姆度規、弗里德曼－勒梅特－罗伯逊－沃尔克度规、米尔恩模型

科學家	爱因斯坦、勒梅特、爱丁顿、史瓦西、闵可夫斯基、克爾、钱德拉塞卡、罗伯逊、弗里德曼、霍金

廣義相對論中的質量此一概念較之於狹義相對論中的質量更為複雜。事實上廣義相對論並沒有對「質量」這一詞彙提供單一的定義，而是提供了許多不同的定義，適用於不同的場合。在一些場合下，廣義相對論中一系統之質量甚至可能是無法定義的。

複習：狹義相對論中的質量[编辑]

主条目：狹義相對論中的質量

在狹義相對論中，一孤立系統之不變質量（此後單純稱為「質量」）可以用此系統的能量與動量來定義：

$m = \frac{\sqrt{E^2 - \left(p c\right)^2}}{c^2}$

其中E 是系統總能量，p是系統總動量，而c 是光速。簡明地說，狹義相對論中一系統的質量為能量-動量四維矢量的範數(norm)。

沿用狹義相對論定義的障礙[编辑]

欲將狹義相對論中的質量定義推廣到廣義相對論，會遇到兩個主要難關。第一個難關在於如何找出一系統之總能量與總動量，情況並不明確。在平直時空只需做積分，將系統各部份之能量-動量四維矢量加總在一起，即可找出整個系統的總能量-動量四維矢量。
但不幸地，如此簡單的程序並無法直接推廣到廣義相對論，以各處的四維矢量存在於不同的切空間，而無法協變式地相加在一起。
第二個難關在於：為了要在廣義相對論中定義質量，必需維持能量是一個守恆量，而已詮釋為時空曲率的「重力場」仍帶有能量，需考慮進去。
但不幸地，廣義相對論中的能量守恆遠不比其他物理學理論中直接。在其他古典理論中，例如牛頓重力、電磁學、流體力學(hydrodynamics)，是可以派定一明確的能量密度值。舉例來說，電場E的能量密度是為 ${1\over 2}\epsilon_0 E^2$ 。
在廣義相對論則不然。事實顯示：一般來說，不可能將「重力能量」派定到一個明確位置上。（參見米斯納(Misner)等人所著，李淑嫻等人所譯之《引力論》(Gravitation)（1973年）第20章第4段）。
欲解決廣義相對論中能量守恆問題的近代手法是完全避免用到「重力場」這一概念，並將能量守恆視為時間平移對稱性(time translation symmetry)的結果。諾特定理(Noether's theorem)當初發展的目的就是特別針對此一問題，每當有時間平移對稱性存在時，則定義了一守恆能量。
然而並非所有系統都有此一要求的時間平移對稱性。對於不具有時間平移對稱性的系統，在廣義相對論中則沒有對於能量的普適定義。

廣義相對論中的質量的數個類型[编辑]

靜態時空中的柯瑪質量(Komar mass)[编辑]

主条目：柯瑪質量

靜態時空的非技術性定義可說為：一時空之度規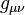 $g_{\mu\nu}\,$ 無一係數是時間函數。黑洞的史瓦西度規及轉動黑洞的克爾度規是靜態時空的常見例子。
照定義，一靜態時空具有時間平移對稱性。技術名詞上，則存在有類時的戚靈向量(Killing vector)。因為此系統有時間平移對稱性，諾特定理保證期會有一守恆能量。又因為一靜態系統也有一良好定義的靜止系，在其中動量會考慮為零值，則定義系統能量也同時定義其質量。在廣義相對論中，這樣的質量稱作是系統的柯瑪質量(Komar mass)。柯瑪質量僅能對靜態系統做定義。
柯瑪質量也可透過一通量積分(flux integral)來定義。這樣的方式類似於高斯定律定義一被一個表面包圍住的電荷是正向電力與面積的乘積。不過，用以定義柯瑪質量的通量積分與用以定義電場的通量積分略有差異——正向力(normal force)並非真實的力，而是在「無限遠處的力」。細節請參見柯瑪質量條目。
上述兩種定義，將柯瑪質量描述為時間平移對稱性者提供了最深層的見解。

phymath999

Friday, July 12, 2013

phymath01 mass of a system in special relativity is the norm of its energy-momentum four vector; otherwise, it is mc.

http://mathsimulationtechnology.wordpress.com/norm-of-a-vector/

Norm of a Vector

目录

複習：狹義相對論中的質量[编辑]

沿用狹義相對論定義的障礙[编辑]

廣義相對論中的質量的數個類型[编辑]

靜態時空中的柯瑪質量(Komar mass)[编辑]

No comments:

Post a Comment